Srinivasa Ramanujan, born on 22 December 1887, was an Indian mathematician who made substantial contributions to Mathematical Analysis, Number Theory, Infinite Series and Continued Fractions, including solutions to mathematical problems then considered unsolvable, despite of being provided with almost no formal theory in Mathematics. During his short life, 22 December 1887 – 26 April 1920, Ramanujan independently compiled nearly 3,900 results. Nearly all his claims have now been proven correct. The Indian government declared 22 December to be National Mathematics Day.
On his birthday, let’s remember a few of his contributions which will surely help the defense aspirants who are appearing with Mathematics.
Defence Exams Online Courses
- NDA 2020 Online Course
- CDS 2020 Online Course
- AFCAT 2020 Online Course
- INET 2020 Online Course
- Territorial Army 2020 Online Course
- Airmen Group X and Y Online Course
In this article we’ll be discussing about 3 such theories of Ramanujan, whose proof will help you as a direct statement:
- The Ramanujan Summation
- Roger-Ramanujan Continued Fraction
- Ramanujan’s Number ‘1729’
Why was he called as “The Man Who Knew Infinity”?
This phrase actually means that Ramanujan was such a natural genius that his contributions to mathematics could reach infinity if he would have lived forever.
Although there can be another reason to call him so. He has given ‘The Ramanujan Summation’ which states that if you add all the natural numbers, that is 1, 2, 3, 4, and so on, all the way to infinity, you will find that it is equal to -1/12.
Let’s look on the proof of this very important result:
The Ramanujan Summation: 1 + 2 + 3 + ⋯ + ∞ = -1/12
Proof: To prove the above statement, let’s prove this by proving two equally crazy claims:
- 1–1+1–1+1–1 ⋯ = 1/2
- 1–2+3–4+5–6⋯ = ¼
Consider A, which is equal to 1–1+1–1+1–1 repeated an infinite number of times
A = 1–1+1–1+1–1⋯
Take away A from 1
1-A=1-(1–1+1–1+1–1⋯)
1-A=1–1+1–1+1–1+1⋯
1-A =A
1-A+A=A+A
1 = 2A
1/2 = A
We move onto proving (2.): 1–2+3–4+5–6⋯ = 1/4
Let the series B =1–2+3–4+5–6⋯ and subtract B from A
A-B = (1–1+1–1+1–1⋯) — (1–2+3–4+5–6⋯)
A-B = (1–1+1–1+1–1⋯) — 1+2–3+4–5+6⋯
Then we shuffle the terms around a little bit, and we see another pattern emerge.
A-B = (1–1) + (–1+2) +(1–3) + (–1+4) + (1–5) + (–1+6) ⋯
A-B = 0+1–2+3–4+5⋯
A-B = B
A = 2B
1/2 = 2B
1/4 = B
Once again, we start by letting the series C = 1+2+3+4+5+6⋯,
subtract C from B.
B-C = (1–2+3–4+5–6⋯) -(1+2+3+4+5+6⋯)
B-C = (1-2+3-4+5-6⋯)-1-2-3-4-5-6⋯
B-C = (1-1) + (-2-2) + (3-3) + (-4-4) + (5-5) + (-6-6) ⋯
B-C = 0-4+0-8+0-12⋯
B-C = -4-8-12⋯
B-C = -4(1+2+3) ⋯
B-C = -4C
B = -3C
And since we have a value for B=1/4, we simply put that value in and we get our result:
1/4 = -3C
1/-12 = C or C = -1/12
Hence proved!
Roger-Ramanujan Continued Fraction:
The Rogers–Ramanujan continued fraction is a continued fraction discovered by Rogers and independently by Srinivasa Ramanujan, and closely related to the Rogers- Ramanujan Identities.
Continued fraction:
A fraction which contains an addition or subtraction-based fraction either in numerator or in denominator or in both is termed as continued fraction.
A finite continued fraction is represented as follows:

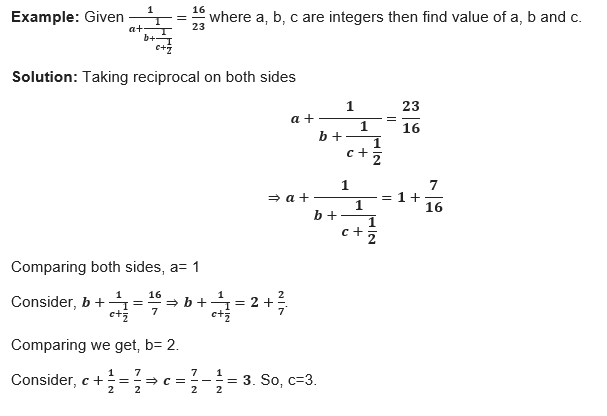
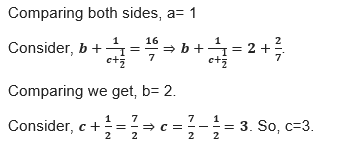
Ramanujan’s Number ‘1729’:
1729 is known as Hardy-Ramanujan number.
Hardy related his and Ramanujan’s conversation as:
“I remember once going to see him when he was ill at Putney. I had ridden in taxicab number ‘1729’ and remarked that the number seemed to me rather a dull one, and that I hoped it was not an unfavorable omen. “No,” he replied, “it is a very interesting number; it is the smallest number expressible as the sum of two cubes in two different ways.”
The two different ways are:
1729 = 13 + 123 = 93 + 103
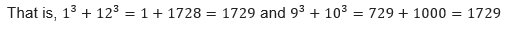
So, isn’t his observation outstanding!
So, I hope all these will be remembered by you as results. It’ll be helpful for you in some or the other way, that’s for sure! Find my lectures on SSBCrackExams, where you’ll find real-time applications of all of the above in the form of questions that can be asked in your examination.



















Hi hello